Indian Antiquary Vol 20

SR No.: 032512
Original Sutra Author: N/A
Author: John Faithfull Fleet, Richard Carnac Temple
Publisher: Swati Publications
Publication Year: 1984
Total Pages: 486
Language: English
Classification: Book_English
File Size: 21 MB

Page 73

FEBRUARY, 1891.)
THE BURMESE SYSTEM OF ARITHMETIC.
63
1
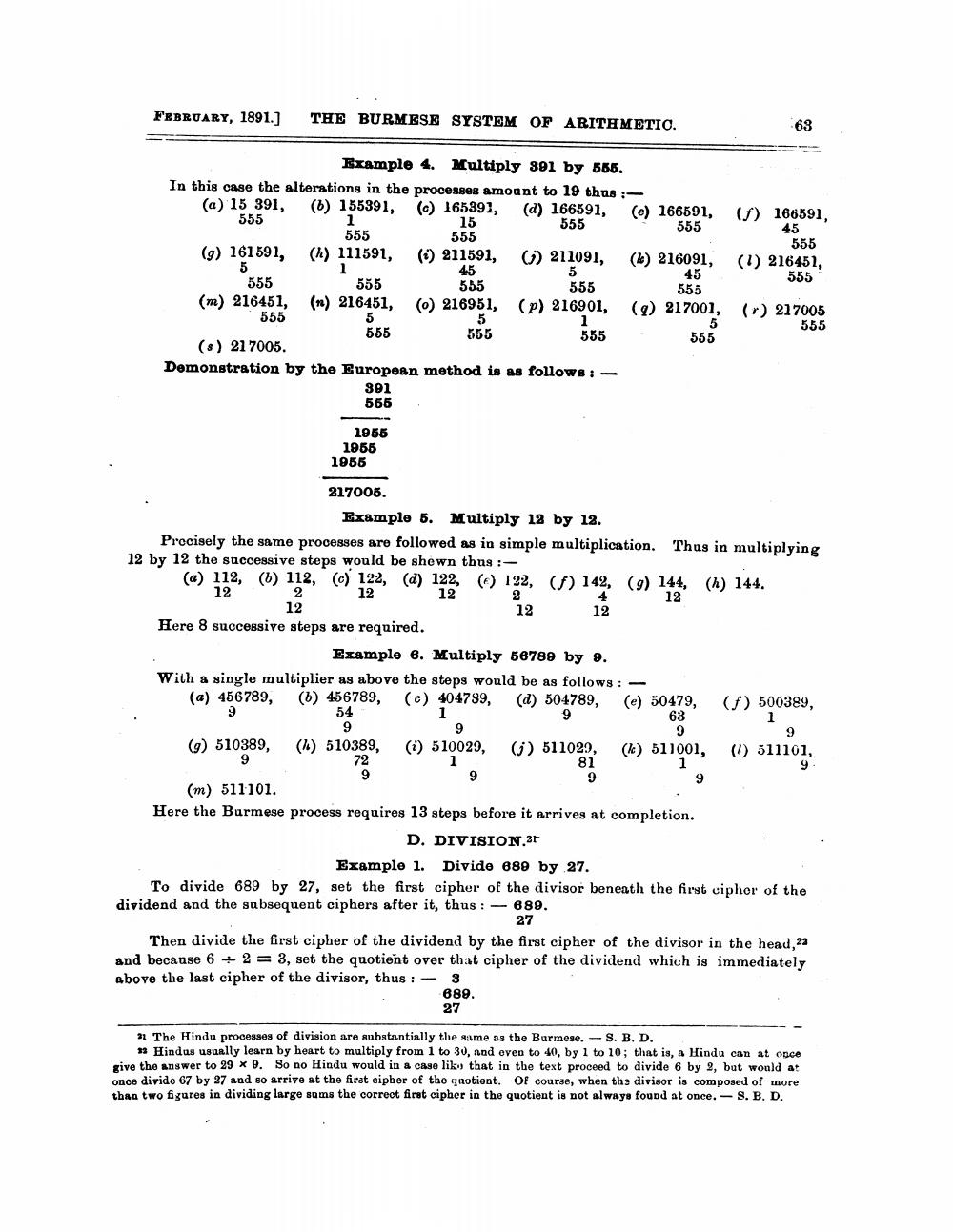
Example 4. Multiply 391 by 566. In this case the alterations in the processes amount to 19 thus :(a) 15 391, (6) 155391, (c) 165391, (d) 166591, (e) 166591, 555
15 555
555 555
555 (g) 161591, (h) 111591, (0) 211591, () 211091, (k) 216091,
45 555 555 555 555
555 (m) 216451, (+) 216451, (0) 216951, (p) 216901, () 217001, 555 555 555
555 (5) 217005. Demonstration by the European method is as follows:
891 555
() 166591,
45
555 (1) 216451,
555
45
(v) 217005
555
1966 1955 1956
2
2
217006.
Example 5. Multiply 12 by 12. Precisely the same processes are followed as in simple multiplication. Thus in multiplying 12 by 12 the successive steps would be shewn thus :(@) 112, (b) 112, () 122, (d) 122 (6) 122, (1) 142, (g) 144, (h) 144.
12
12 12
12 12 Here 8 successive steps are required.
Example 6. Multiply 56789 by 9. With a single multiplier as above the steps would be as follows:(a) 456789, (6) 456789, (c) 404789, (d) 504789, (e) 50479, (f) 500389,
54
1 (g) 510389, (1) 510389, (i) 510029, (j) 511029, (k) 511001, (1) 311101,
63
9
(m) 511101. Here the Burmese process requires 13 steps before it arrives at completion.
D. DIVISION.2
Example 1. Divide 889 by 27. To divide 689 by 27, set the first cipher of the divisor beneath the first cipher of the dividend and the subsequent ciphers after it, thus: - 689.
27 Then divide the first cipher of the dividend by the first cipher of the divisor in the head.23 and because 6 + 2 = 3, set the quotient over that cipher of the dividend which is immediately above the last cipher of the divisor, thus: - 3
689.
27
* The Hindu processes of division are substantially the same as the Burmese. - S. B. D.
Hindus usually learn by heart to multiply from 1 to 30, and even to 40, by 1 to 10; that is, a Hindu can at once give the answer to 29 x 9. So no Hindu would in a case lik, that in the text proceed to divide 6 by 2, but would at once divide 67 by 27 and so arrive at the first cipher of the quotient. Of course, when the divisor is composed of more than two figures in dividing large sums the correct firat cipher in the quotient is not always found at once. -S. B. D.